2024 PSLE Mathematics Paper 1 & 2 Review: What You Need to Know

The 2024 PSLE Math paper was, by most accounts, an eas­i­er one com­pared to pre­vi­ous years. But as always, suc­cess wasn’t just about know­ing formulas—it was about spot­ting pat­terns, apply­ing the right heuris­tics, and man­ag­ing time effec­tive­ly. Let’s break down the key trends that sur­faced in this year’s exam and see how cer­tain tech­niques would have ensured stu­dents solved the ques­tions effi­cient­ly.

A Familiar Question Type Appeared FOUR Times

Across Paper 1 and Paper 2, one par­tic­u­lar type of ques­tion appeared not once, not twice, but four times! This con­cept showed up in:

• Paper 1, Book­let A: Ques­tion 13
• Paper 2: Ques­tions 10, 15, and 17

What did these ques­tions test? The abil­i­ty to form equa­tions and solve area and perime­ter-relat­ed problems—a clas­sic con­cept, but one that required stu­dents to think crit­i­cal­ly. Let’s break them down.

Paper 1, Question 13: Area and Perimeter

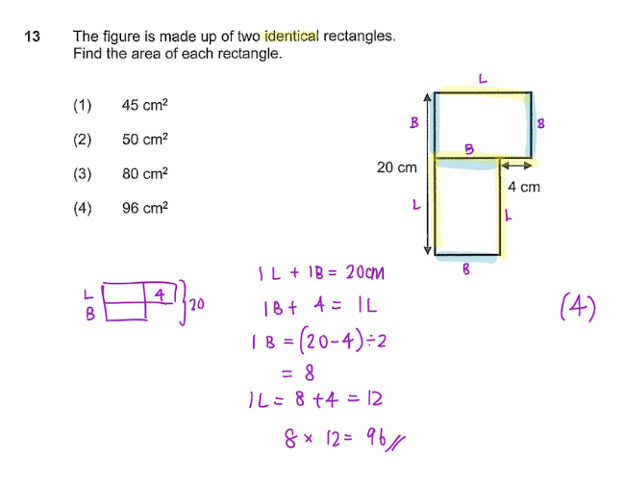
In this ques­tion, stu­dents were giv­en a key piece of infor­ma­tion: 1 breadth and 1 length add up to 20 cm. To find the area of the rec­tan­gle, both dimen­sions were need­ed.

The dia­gram also pro­vid­ed a cru­cial clue: the length is 4 cm longer than the breadth. With that in mind:

There­fore, 2 breadths + 4cm = 20cm

2 breadths = 16 cm

1 breadth = 8cm 

 1 length = 12cm

The area of 1 rec­tan­gle is 12 cm x 8 cm, which gives us 96 cm². 

A straight­for­ward approach for those who iden­ti­fied the rela­tion­ships quick­ly!

Question 10 of Paper 2

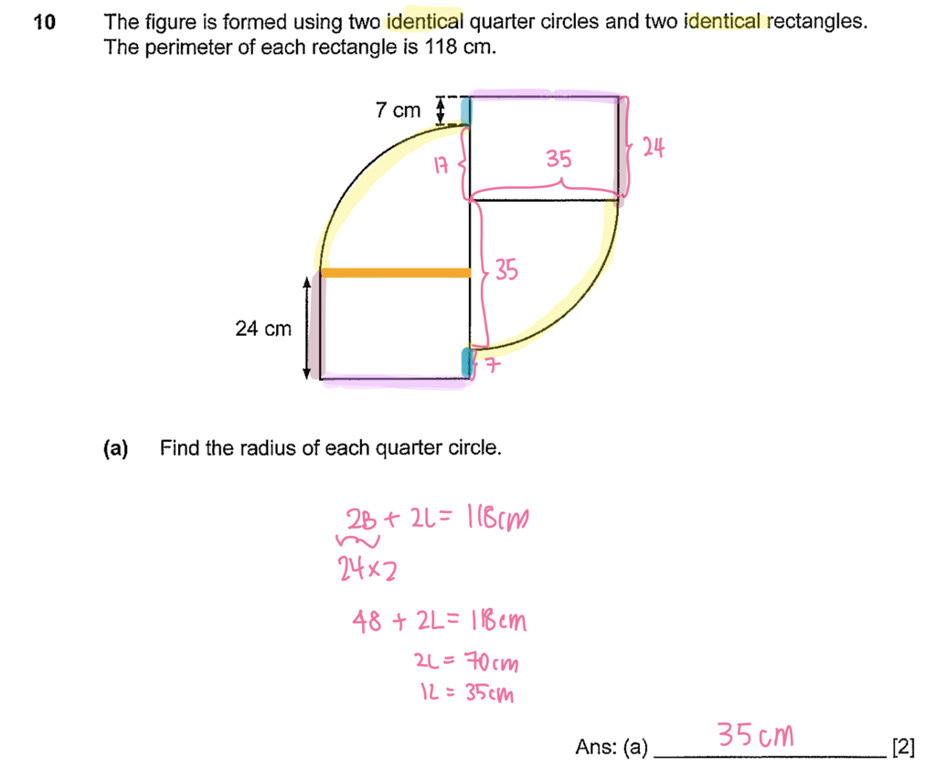
This ques­tion test­ed the abil­i­ty to con­nect dif­fer­ent geo­met­ric prop­er­ties. The key obser­va­tion?

If you look at the orange line, you will see that the radius of the quar­ter-cir­cle is the length of the rec­tan­gle. The ques­tion gave the perime­ter of the rec­tan­gle, and that is to help us to solve for the radius of the quar­ter-cir­cle. 

Since the breadth of the rec­tan­gle is giv­en, we can eas­i­ly find the length of the rec­tan­gle. 

1B = 24cm

2B = 48cm

2B + 2L = 118cm

48cm + 2L = 118cm

2L = 70cm

1L = 35cm

Since A and B stacked up togeth­er and C and D stacked up togeth­er are the same length, this means that if I shift­ed C and D upwards by 7cm, it would cre­ate the same 7cm gap at the bot­tom. Both blue lines in the dia­gram are 7cm each. 

The yel­low lines are the arcs of the quar­ter-cir­cles. As the quar­ter-cir­cles have the same radius, this means that they are the same size. When placed togeth­er, they will form a semi­cir­cle. Hence, the yel­low lines togeth­er form the arc of a semi­cir­cle. 

The for­mu­la for find­ing the arc of a semi­cir­cle is half of pi x diam­e­ter.

To find the length of the yel­low lines, we take ¹⁄₂ × ²²⁄₇ × 70cm = 110cm

The perime­ter of the fig­ure is 110 + (2 × 7) + (2 × 24) + (2 × 35) = 242cm.

Question 15 of Paper 2

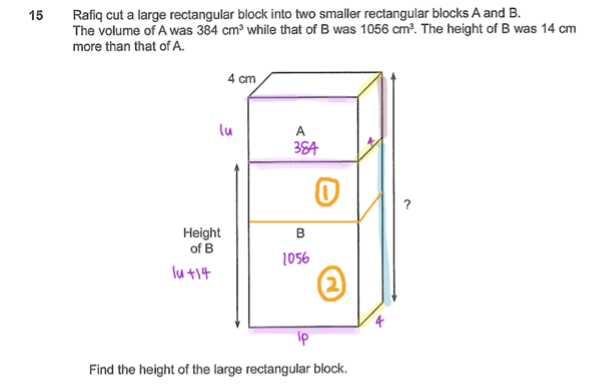
In this prob­lem, two rec­tan­gu­lar blocks (A and B) shared the same base area but had dif­fer­ent heights. Know­ing that L × B × H = Vol­ume, and L × B = base area and their respec­tive vol­umes, we can make use of the vol­ume and base area to find the height. 

To make it a bit more visu­al and eas­i­er to under­stand, block B was cut out into 2 sec­tions such that one of them has the same height as block A, and the oth­er block will have a height of 14 cm. You can refer to these new blocks as block 1 and block 2. 

Do you then see that Block 1 and Block A have the same dimen­sions? They there­fore have the same vol­ume. Block 2 has a height of 14 cm. The vol­ume of Block 2 is there­fore the dif­fer­ence in vol­ume between Blocks A and B. 

Block 2 —> 1056 — 384 = 672cm 

Base area —> 672 ÷ 14 = 48cm²

Now that we have the base area and the vol­ume of Block A, 

we can find out the height of Block A. 

Height of Block A —> 384 ÷ 48 = 8cm

The height of the rec­tan­gu­lar block is 8 + 8 + 14 = 30cm

A clas­sic case where break­ing the prob­lem into small­er steps made all the dif­fer­ence.

Question 17 of Paper 2

When­ev­er it comes to ques­tions that involve fold­ing paper, we tell stu­dents that fold­ing paper would cre­ate dupli­cate angles and dupli­cate shapes. There­fore, what­ev­er that was cut off from the orig­i­nal rec­tan­gu­lar piece of paper was 2 iden­ti­cal tri­an­gles that each was the size of tri­an­gle ABC. 

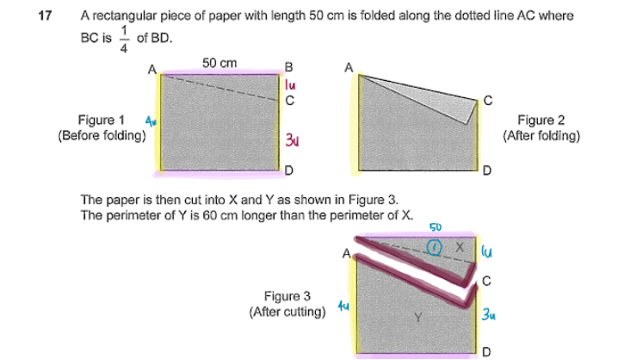
It is impor­tant to note that line BC is ¹⁄₄ of line BD. 

Since line BD is 4u, then BC will be 1u and line CD is 3u. 

The infor­ma­tion in the ques­tion focused on the dif­fer­ence in perime­ter between the two shapes. To make it obvi­ous where the addi­tion­al 60cm in perime­ter came from, it would be help­ful to high­light iden­ti­cal lines in X and Y. 

The brown lines are the same length. The pur­ple lines are 50cm each. The dif­fer­ence in length comes from the yel­low lines. In X, the yel­low line is 1u long. In Y, the yel­low lines add up to 7u. 

There­fore, 6u = 60 cm

1u =10cm

4u = 40cm

Line BD is 40cm. 

To quick­ly find the area of part Y, we take the area of the rec­tan­gle and minus away the 2 iden­ti­cal tri­an­gles that were cut out (Part X). 

A Twist on the Classic Sets Question Type

This year, Ques­tion 28 in Paper 1 and Ques­tion 3 in Paper 2 fea­tured sets and num­ber sequences in a slight­ly dif­fer­ent way.

Paper 1, Question 28: Reversed Number Pattern Question

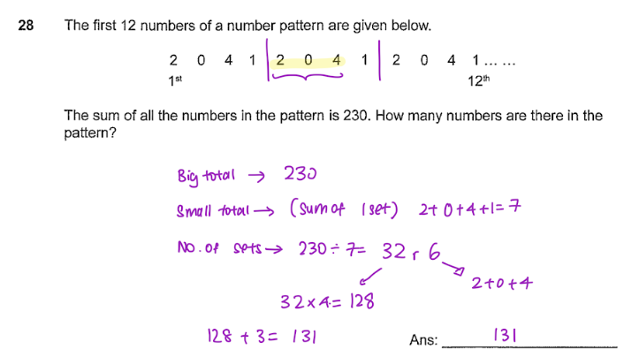
Instead of ask­ing for the sum of the first 131 num­bers, 2024’s PSLE flipped the ques­tion: Giv­en the sum, find the num­ber of terms in the sequence.

As with most Sets ques­tion types, we start with the same three steps of 

1. Big Total / Dif­fer­ence
2. Small Total / Dif­fer­ence
3. No. of Sets

The big total / dif­fer­ence is always giv­en in the ques­tion. In this case, the big total is 230. Based on the num­ber sequence, the repeat­ed pat­tern is 2, 0, 4, 1. This means that there are 4 dig­its in one set, and the sum of 1 set is 2 + 0 + 4 + 1 = 7.

How many 7s are there in 230? We always take Big Total / Small Total to find the num­ber of sets. As you can see, there is a max­i­mum of 32 sets, with a remain­der of 6. 

Since 1 set con­sists of 4 dig­its, 32 sets would con­sist of 32 × 4 = 128 dig­its. To achieve a sum of 6, we need to con­tin­ue with the sequence and include 3 more dig­its. The dig­its 2 + 0 + 4 in the sequence would give us a sum of 6. 

There­fore, the total num­ber of dig­its would be 128 + 3 = 131.

If you add up the 1st 131 dig­its of the num­ber pat­tern, you will get a sum of 230.

Paper 2, Question 3: LCM and Coin Count Problem

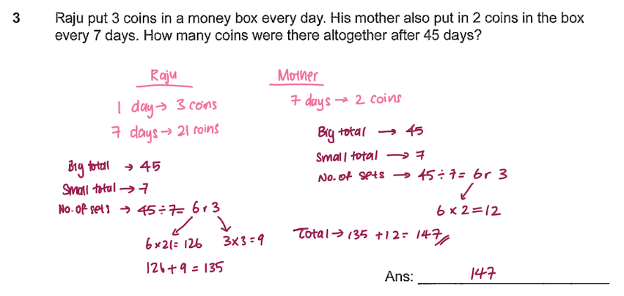
This sets ques­tion is inter­est­ing because it is not imme­di­ate­ly appar­ent that you would have to find the LCM of 1 day and 7 days. Doing so would help with count­ing how many coins they had after 45 days. 

In 7 days, Raju would have put in 21 coins and his moth­er would have put in 2 coins. 

This means that every 7 days, there would be 23 coins added to the mon­ey box. 

Again, we start with the same three steps: 

1. Big Total / Dif­fer­ence
2. Small Total / Dif­fer­ence
3. No. of sets

The big total giv­en in the ques­tion is 45 days. In a set, we know that every 7 days, there would be 23 coins added. Since the big total giv­en is the num­ber of days, the small total is 7 days, and not 23 coins. 

How many “7 days” do we have in a peri­od of 45 days?

When we take the big total divid­ed by the small total, we will see that we have a max­i­mum of 6 sets, with a remain­der of 3 days. 

Since 1 set con­sists of 23 coins, 6 sets would con­sist of 6 × 23 = 138.

As for the next 3 days, Raju would have put in 3 × 3 = 9 more coins. 

Since his moth­er only puts in 2 coins every 7 days, she wouldn’t have put in any coin in a peri­od of 3 days. 

There­fore, the total num­ber coins in the box after 45 days would be 138 + 9 =147

Paper 1, Questions 14 and 30: Information-Heavy Problems

Two ques­tions in Paper 1—Questions 14 and 30—stood out for the sheer amount of data they pro­vid­ed. The chal­lenge? Extract­ing rel­e­vant details, set­ting up the right approach, and solv­ing sys­tem­at­i­cal­ly.

P, Q, R are con­tain­ers with the same capac­i­ty. Based on the frac­tion in the ques­tion, this means that each con­tain­er has a capac­i­ty of 5u. Q and R were ¹⁄₅ filled and P was com­plete­ly filled. This means that 1 and ²⁄₅ con­tain­ers were filled.

1 ²⁄₅ = ⁷⁄₅

To have the same amount of water in each con­tain­er, it will be ⁷⁄₅ ÷ 3 

When it comes to divid­ing frac­tions, stu­dents are taught to KCF (Keep-Change-Flip) 

Keep the first frac­tion in the equa­tion the way it is, change the divi­sion sym­bol to mul­ti­pli­ca­tion, and flip the sec­ond frac­tion over such that the numer­a­tor is now the denom­i­na­tor and the denom­i­na­tor is now the numer­a­tor. 

After sim­pli­fy­ing and mul­ti­ply­ing, we will see that each con­tain­er should be ⁷⁄₁₅ full in the end. 

Since P was ini­tial­ly full, and end­ed up being only ⁷⁄₁₅ full in the end, this means that ⁸⁄₁₅ of water in P was poured out.

Question 30 of Paper 1

First of all, both boards start­ed with a dif­fer­ent num­ber of objects on them.

Sec­ond­ly, each stu­dent pinned EITHER hearts or stars. 

Third­ly, the num­ber of hearts and stars on the boards was equal. 

Since there were already 22 paper hearts on the board, after the first child sticks on 5 paper hearts, there would be 27. If the next child sticks on anoth­er 5 paper hearts, there would be 32. 

There­fore, we are look­ing at Mul­ti­ples of 5 + 22. 

For the paper stars, we start off with 2 on the board. After the first child sticks on 8 paper stars, there would be 10, If the next child sticks on anoth­er 8 paper stars, there would be 18. 

There­fore, we are look­ing at Mul­ti­ples of 8 + 2.

The ques­tion stat­ed that the num­ber of paper hearts and paper stars was equal in the end. 

After list­ing out a few, we see that 42 is the low­est com­mon num­ber between the num­ber of stars and hearts. 

In order to have 42 paper hearts on the board, 4 chil­dren stuck a total of 20 hearts.

In order to have 42 paper stars on the board, 5 chil­dren stuck a total of 40 stars.

There­fore, the num­ber of chil­dren in the ques­tion is: 

4 chil­dren who pinned hearts + 5 chil­dren who pinned stars = 9 chil­dren in total.

Final Thoughts: Why 2024’s PSLE Math Paper Was Manageable

This year’s PSLE Math paper reaf­firmed a clear trend: suc­cess hinges on a strong grasp of heuris­tics and effi­cient prob­lem-solv­ing tech­niques. If stu­dents knew their heuris­tics con­cepts and applied them effec­tive­ly, this year’s PSLE Math paper was actu­al­ly quite man­age­able. The abil­i­ty to quick­ly recog­nise ques­tion types and apply the right strate­gies made all the dif­fer­ence.

Mov­ing for­ward, stu­dents must strength­en their con­cep­tu­al under­stand­ing of area and perime­ter, sets, and visu­al­i­sa­tion-based geom­e­try ques­tions—all of which fea­tured promi­nent­ly in this year’s exam. Addi­tion­al­ly, mas­ter­ing short­cut tech­niques like BCA / Equat­ing Numer­a­tors can sig­nif­i­cant­ly reduce solv­ing time for com­plex prob­lem sums.

At Think Teach, we make note of these PSLE trends and drill stu­dents in ques­tions that have been appear­ing fre­quent­ly based on school papers as well as the PSLE of recent years. By equip­ping stu­dents with Short­er-Faster-Bet­ter Meth­ods, we empha­sise on ques­tion iden­ti­fi­ca­tion before guid­ing them to apply the most time effi­cient tech­niques to approach these ques­tions. 

Ulti­mate­ly, excelling in PSLE Math isn’t about luck—it’s about strate­gic prepa­ra­tion, pat­tern recog­ni­tion, and apply­ing the right tech­niques with con­fi­dence.

If you would like for your child to learn this approach or any of the tech­niques men­tioned, reg­is­ter your inter­est via this link: https://thinkteachacademy.com/shop/tr-fiona/